Boson stars are a remarkable type of self-gravitating soliton, which admits multipolar configurations. The simplest non-spherical stars, but still static, are the dipoles, but are they dynamically stable? In the paper "Self-interacting dipolar boson stars and their dynamics", it is shown self-interactions help make these dipoles dynamically robust.
Boson stars appear in many guises - see e.g. this Living Review in Relativity. One of these guises is as multipolar stars, the simplest non-spherical case being the dipolar boson stars, which can also be seen as two spherical boson stars (with a certain phase difference) in equilibrium - see the discussion in this paper. These are therefore an illustration of an equilibrium two body problem in General Relativity. But how dynamically robust are these configurations?
In this paper, it was shown that the dipolar boson stars in the simplest model where the scalar field has no-self interactions decay quite fast (e.g. into a spherical boson star). Is there a way to heal this dynamical frailty?
It has been shown in several contexts, e.g. see these papers - [1], [2], [3], [4] - that scalar self-interactions can mitigate dynamical instabilities of excited states of boson stars. In the paper "Self-interacting dipolar boson stars and their dynamics" it is shown this is also the case for dipolar boson stars with a particular type of self-interaction, named dipolar Q-stars.
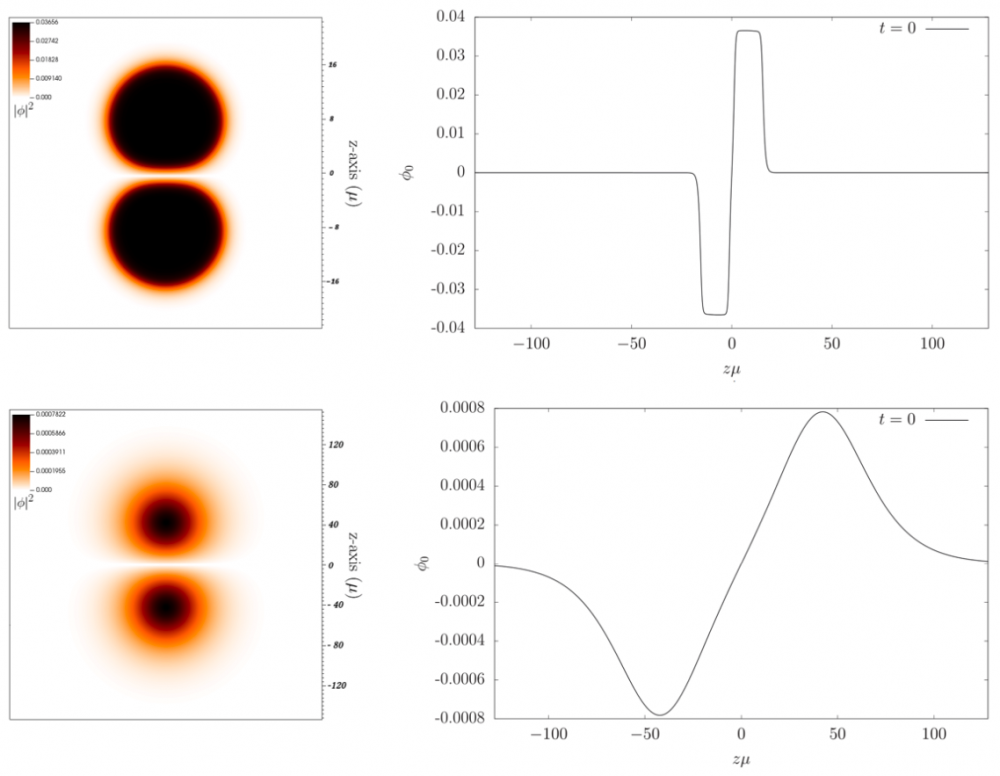
In the following we show some animations of the dynamical evolutions of dipolar Q-stars reported in this paper.
Video 1: Dipolar Q-star beyond the relativistic branch - Solution 1 in the paper - it decays into a black hole.
Video 2: Dipolar Q-star at the threshold of the relativistic branch - Solution 2 in the paper - it decays into a black hole.
Video 3: Dipolar Q-star in the relativistic branch - Solution 6 in the paper - it remains robust.
Video 4: Dipolar Q-star in the relativistic branch - Solution 8 in the paper - it remains robust.
Video 5: Dipolar Q-star in the middle branch - Solution 10 in the paper - it migrates.
Video 6: Dipolar Q-star in the middle branch - Solution 11 in the paper - it migrates.
Video 7: Dipolar Q-star in the Newtonian branch - Solution 12 in the paper - it remains robust.