Gr@v researchers have found that there are only two equilibrium possibilities for the spin of black hole binaries, and that they are similar to the equilibria observed for the spin of the Moon, which was identified by Giovanni Domenico Cassini in 1693. The results were published this week in MNRAS Letters.
Following observations of the Moon, Cassini (1693) established three empirical laws on its rotational motion. The first stated that the rotation rate and the orbital mean motion are synchronous, the second that the angle between Moon’s equator and the ecliptic is constant, and the third that the Moon’s spin axis and the normals to its orbital plane and ecliptic remain coplanar. The observed physical librations are described as departures of the rotational motion from these three equilibrium laws.
Black hole binaries are a system composed of two black holes that orbit each other. Due to gravitational waves emission, the orbit shrinks until the two black holes merge in a larger black hole. During the fusion process, the resulting black hole receives a kick, that can eject it from the galaxy. The recoil velocity depends on the final distribution of the relative spins of the initial black holes before merging. The final spin evolution of black holes is then particularly interesting, since the spin alignment during the inspiral phase can change significantly the distribution of black hole recoil velocities.
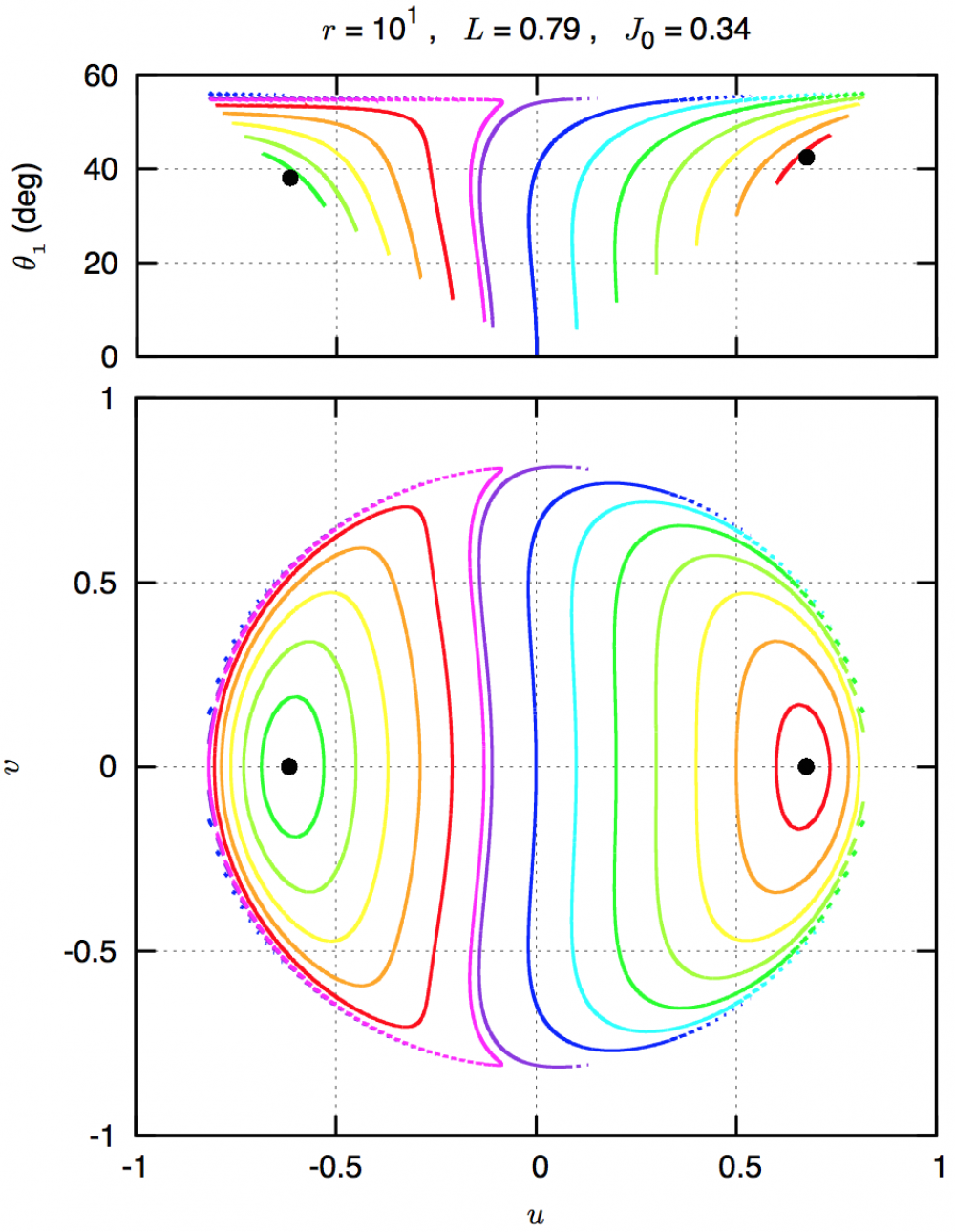
Jeremy Schnittman has found in 2004 that spinning black hole binaries can present stable configurations where the two spin axes and the orbital angular momentum vector remain coplanar. Because of the loss of energy and orbital angular momentum through gravitational radiation reaction, the spins may end in libration around these equilibria. However, one of the most difficult aspects of studying the spinning black hole binary system is the problem of visualising and analysing the orientation of the two spins in an informative way.
Gr@v researchers have now revisited the spin dynamics of black hole binaries using a new analytical method (MNRAS Letters). It was possible to determine all possible configurations for the spins before merging in a simple way. Moreover, Gr@v researchers have identified that there are only two equilibrium possibilities for the spin and that they are similar to the equilibria observed by Cassini for the spin of the Moon in 1693. Therefore, these equilibria states were renamed "Cassini states" for black hole binaries.